Articles

Beautiful black and blue butterflies

by Jonathan Sarfati

Iridescent blue

Some butterflies have the most striking iridescent blue wings, such as the blue morpho (Morpho menelaus) of South America and the male mountain blue don (Papilio ulysses) of northern Australia. (1) How is this striking blue produced? It was long known that the blue was not produced by a pigment, but by some optical effects.

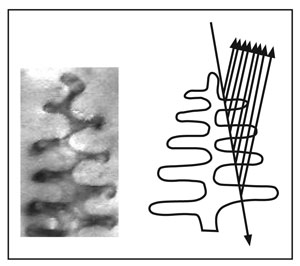
Figure 1. Left: morpho butterfly multilayer structure under microscope. Right: how light reflects from different surfaces. After Interference in multilayers, Exeter University, 25 January 2005.

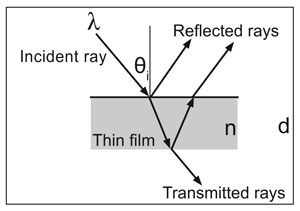
Figure 2. If the difference in path length of the reflected ray is a whole number of a particular wavelength, then constructive interference (see fig. 3) will occur for that wavelength—that is, if the thickness d satisfies the formula mλ = 2ndcosθ I , where m is the order of diffraction, n the refractive index, λ the wavelength in air, and θ I the angle of incidence. After Interference in multilayers , Exeter University, 25 January 2005

In 2001, Pete Vukusic of the University of Exeter found that the blueness in butterflies is caused by optical interference.2 The scales have multilayering that reflects light waves so that they travel different distances (see fig. 1, right). The point is that with some wavelengths, the light reflected from top and bottom surface will have a travel distance of a whole number of wavelengths, so the crests align with other crests (see fig. 2). This is called constructive interference, and makes this colour (blue in this butterfly) much brighter and purer (see fig. 3). To produce a particular colour, the layer thickness must be accurate to within about 0.05 ,m. The opposite is destructive interference, where the wave crests align with troughs, cancelling each other out (fig. 4).

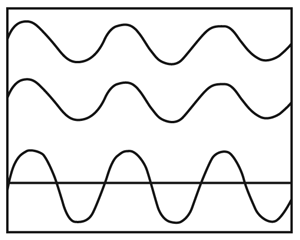
Figure 3. Constructive interference. When two waves are in phase, i.e. the crests line up with crests and the troughs line up with troughs, their intensities reinforce each other. The bottom wave with the greater amplitude is the result.

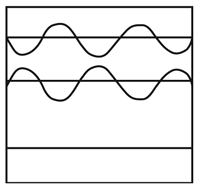
Figure 4. Destructive interference. When two waves are totally out of phase, i.e. the crests line up with troughs, they cancel each other out. The bottom line, with no wave at all, is the result.

Scientists learn from ‘nature’

Sometimes different colours can be seen from interference, depending on the angle. That is, at a shallower angle, the difference in path length becomes greater, so higher wave lengths constructively interfere. Thus, ordinary diffraction gratings produce pretty rainbow effects but not pure colours. But the butterfly wing has now inspired imitation structures that appear intensely blue from a wide range of angles. Mool Gupta, director of the Applied Research Center at Old Dominion University in Virginia, US, said:

“We started examining butterfly wings using electron microscopy and we learned how complex the structures are and difficult to fabricate. So we came up with a new approach of using micro-gratings with random orientation as a means to achieve colour with a wider viewing angle.” (3)

So they made a honey-comb–like array of tiny hexagons, each of which had diffraction gratings with the grooves in different directions (see fig. 5, below). They used a technique called electron beam lithography (EBL). The result was a structure that looked blue from a viewing range of 16–90°. (4)

In future, these new techniques could be used in display devices, and to replace paints for coating surfaces, thereby producing better colours. They would avoid the problems of chemical waste in production of pigments and dyes. (5)

Blue budgies

The bright blue colour of some budgie feathers is also due to interference. But in this case it’s caused by the spongy structure of the keratin, the protein that feathers (and skin and nails) are made of.6 The dazzling colours of the peacock tail are also caused by interference. (7)

Blacker than black

Black pigments appear black because they absorb all frequencies of visible light. However, this is not perfect, and some light scatters back. A notable example is the moon—it looks bright silvery-white from Earth, but its surface largely comprises the black rock basalt.

A well-known experiment can demonstrate the imperfection of black paints. Take an enclosed cardboard shoebox and paint it the blackest black possible. Then cut a small hole in it, 1–2 mm. This hole should appear far blacker than even the blackest paint. While the paint scatters some light back, light entering the hole hardly ever escapes. Indeed, that’s why the pupil of the eye is so black—it’s a hole to let light in, and it doesn’t get out again.

More recently, Dr Vukusic has shown that the black outline of the blue don is also caused by an optical light-trapping design. (8) This special blackness is almost twice that which could be achieved by pigment alone, and causes the bright blue to stand out even more.

The scales are covered in tiny pits, about a micron across, that form a honeycomb-like array. (9) These scales have a high refractive index, so they take advantage of total internal reflection. That is, the light enters the material, but whenever the light meets another part of the surface, instead of crossing, it is reflected back into the material. (Optical fibres work that way, including the natural ones of the Venus flower basket sponge. (10) Since hardly any light can escape from the wings into the eyes, they appear very black.

Removing the refractive effect

Dr Vukusic’s success in working out the blue don’s light-trapping design came about because he wanted to see what would happen if he could somehow remove the refractive effect. But how can one remove that effect?

Light refraction (i.e. change of direction) and reflection can occur at surfaces between substances where the speed of light inside each substance is different, e.g. light travels more slowly through water than through air, so water’s refractive index is higher. 11 This is why, when one immerses a straight stick into a swimming pool, it looks ‘bent’ at the surface of the water. If the different substances have the same index, then the light behaves as if it is not changing between substances at all, so there is little reflection or refraction.

This can be demonstrated with a pane of frosted glass. This is deliberately made with a rough surface so that light scatters in all directions at the glass–air interface, making a clear image impossible. However, an image IS possible if this scattering can be eliminated. One way is to smear a viscous liquid with about the same refractive index as glass onto the frosted glass, then cover it with smooth glass. With hardly any reflection or refraction from the frosted glass surface into the liquid, it is now possible to see a clear image through it. Dr Vukusic applied the same principle to the butterfly. He immersed the wings in bromoform (CHBr3), which has about the same refractive index as the wing tissue. As a result, the wings could only absorb just over 50% of the light, while in air, they absorbed over 90%.

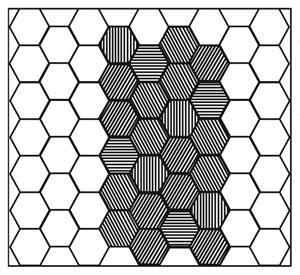
Figure 5. Artificial butterfly colour. The grooves in each hexagon are 125 nm deep and 220 nm wide. After Mool Gupta, Grating Structure, 25 January 2005.

More biomimetics

The chemical engineer Richard Brown of Britain’s National Physical Laboratory in Teddington, near London, has used this principle to make ‘Super Black’. This is a nickel-phosphorus alloy coating with pits that also exploit light refraction. This absorbs 99.7% of the light.

This is yet another example of how the design in nature has inspired human engineers. Dr Vukusic says, ‘Biomimetics is growing in popularity. Wherever we can we should take cues from nature.’ What this really should mean is copying the genius of the Creator of nature.

References

1. Cardno, S. and Wieland, C., Mr Butterflies, Creation 19(2):44–46, 1997.

2. Vukusic, P., Sambles, R., Lawrence, C. and Wakely, G., Sculpted-multilayer optical effects in two species of Papilio butterfly, Applied Optics 40(7):1116–1125, 2001.

3. Graydon, O., Blue microstructures mimic nature, Optics.org, 8 December 2003.

4. Wong, T.-H., Gupta, M.C., Robins, B. and Levendusky, T.L., Color generation in butterfly wings and fabrication of such structures, Optics Letters 28(23)2342–2344, 2003.

5. Lerner, E.J., Butterfly blues, The Industrial Physicist, , Briefs, April 2004.

6. Prum, R.O., Torres R.H., Williamson S. and Dyck J., Coherent light scattering by blue feather barbs, Nature 396(6706):28–29, 1998; Two-dimensional fourier analyses of the spongy medullary keratin of structurally coloured feather barbs, Proceedings of the Royal Society London B 266:13–22, 1999. Burgess, S., The beauty of the peacock tail and the problems with the theory of sexual selection, Journal of Creation 15(2)94–102, 2001.

7. Butterflies plumb the depths of blackness with a trick of the light, New Scientist 181(2433):18, 2004.

8. Hopkin, M., Butterflies boast ultrablack wings: Insects use optical trick to get the blackest black out of dark pigments, Nature science update, 28 January 2004.

9. Sarfati, J., Fantastic fibre-optics: Sponge’s super spicules, Creation 26(2):52, 2004. Return to text.

10. The refractive index (n) of a substance is given by n = c/v, where c is the speed of light in a vacuum, and v the speed of light in the substance.

http://www.creationontheweb.com/content/view/4317

Used by permission of Creation Ministries International: www.creationontheweb,com.